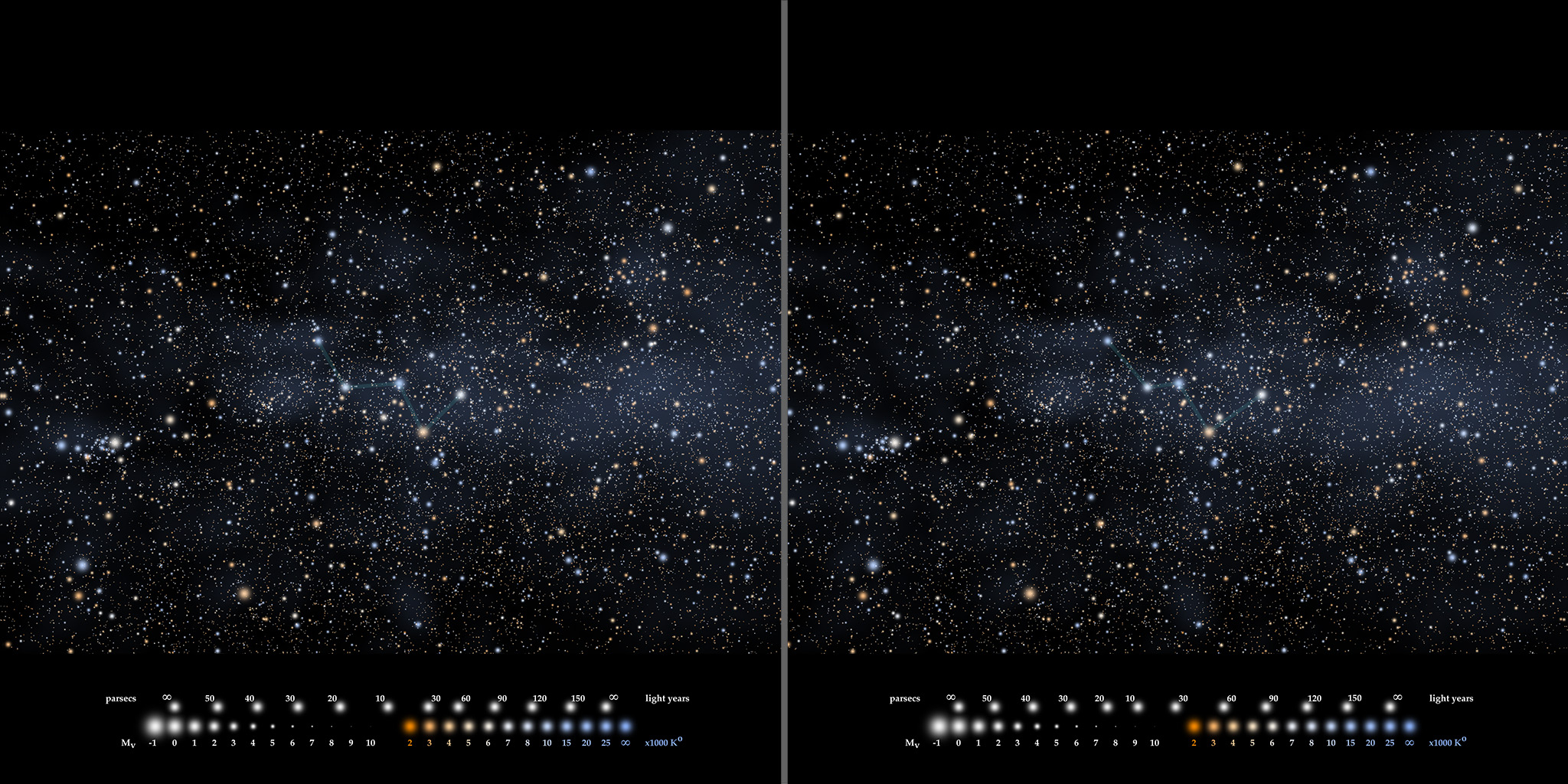
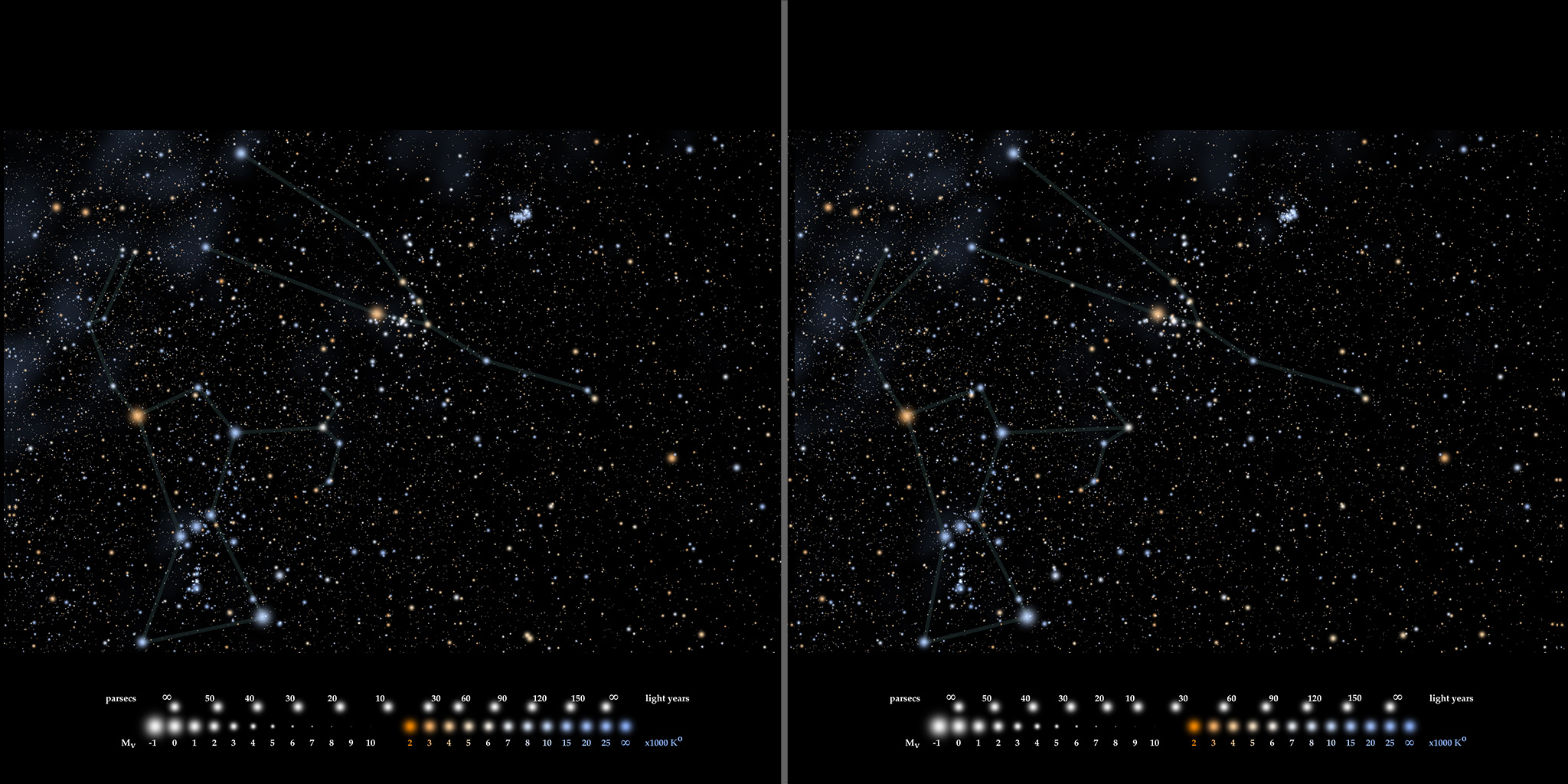
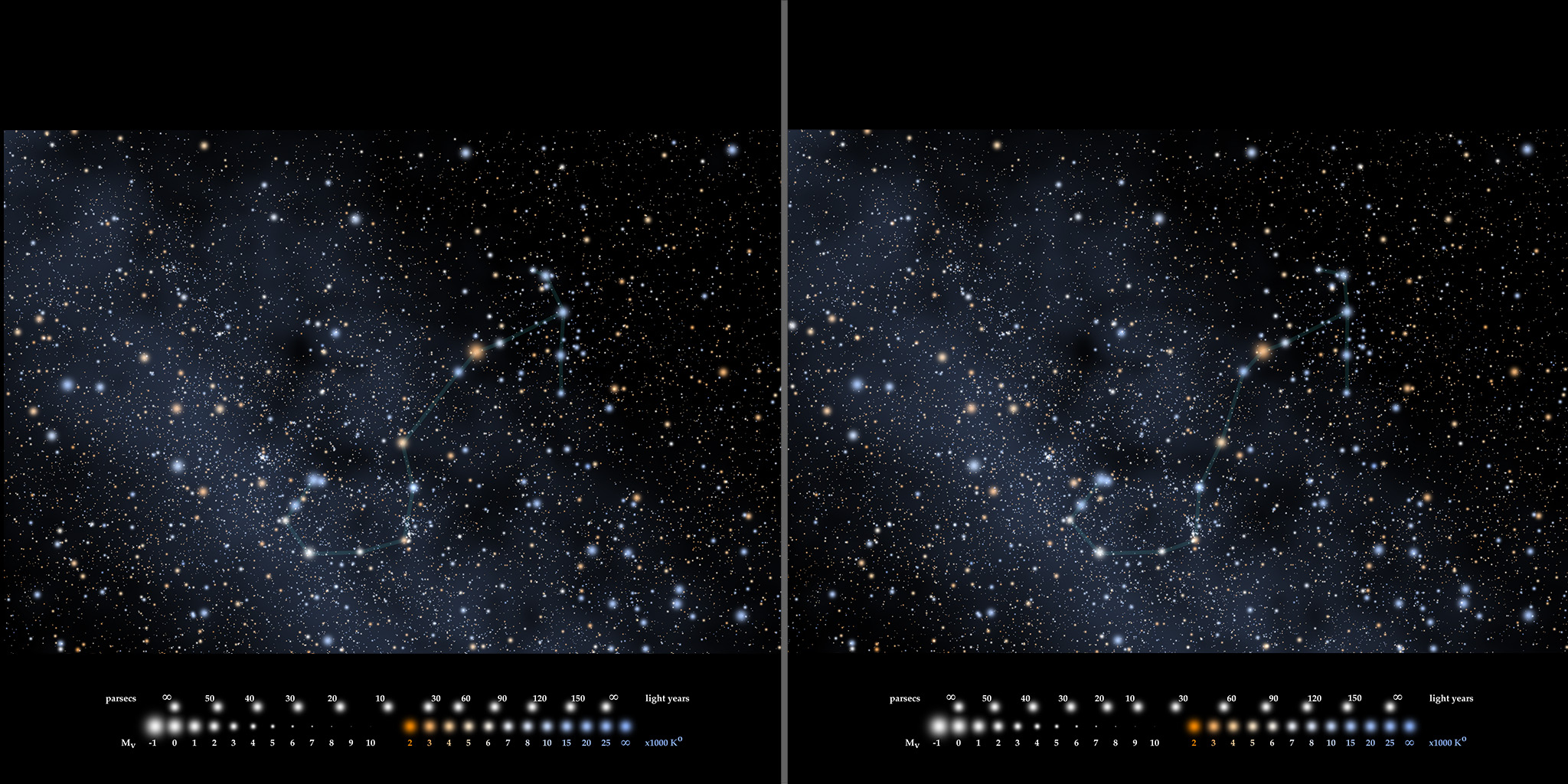
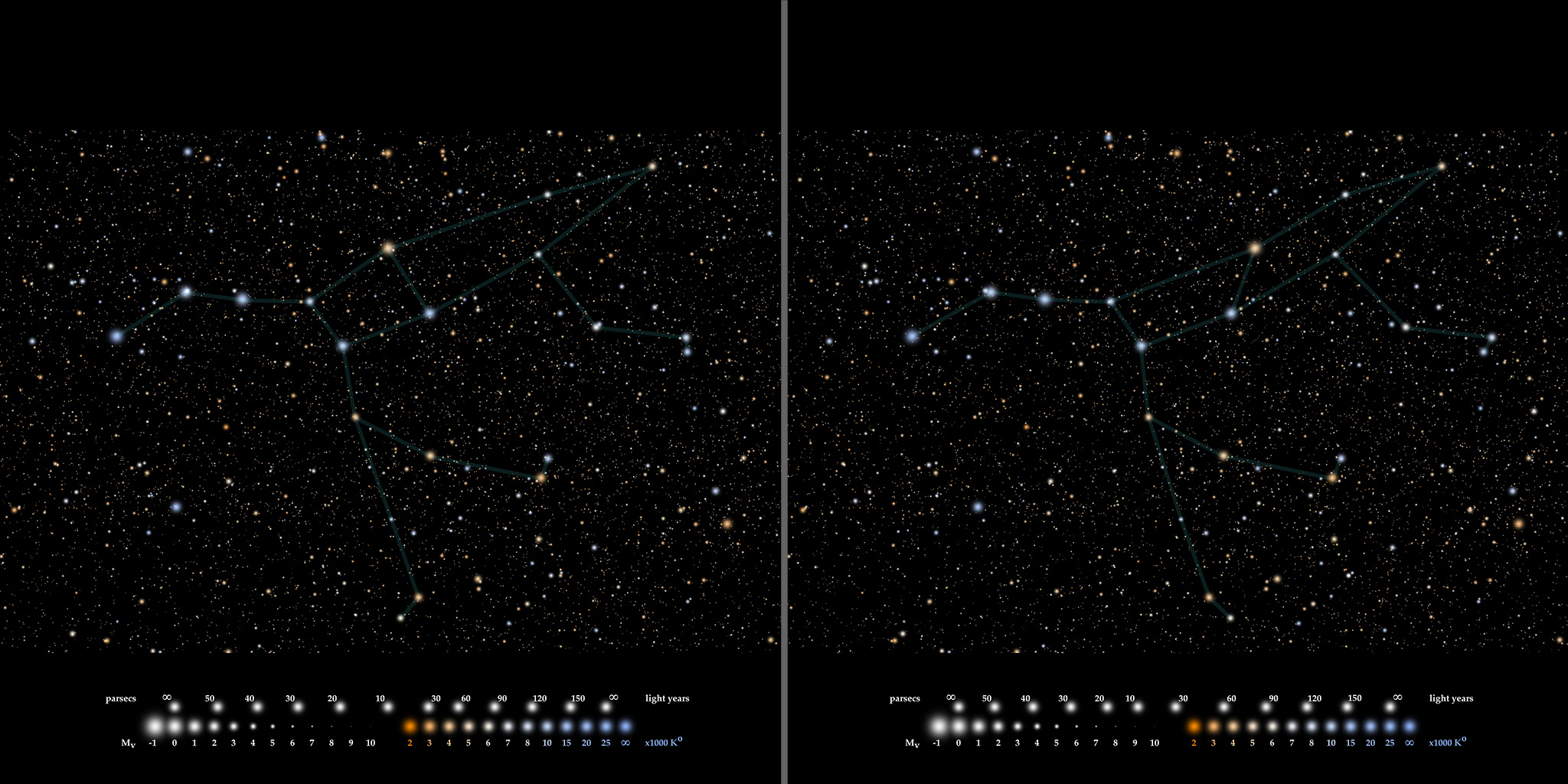
Here is the set of planets presented for cross-eye or wall-eye viewing all on one page. You can click on them to expand and examine them in detail at their full resolution. Cross-eye images are first, followed by the wall-eyed pairs.


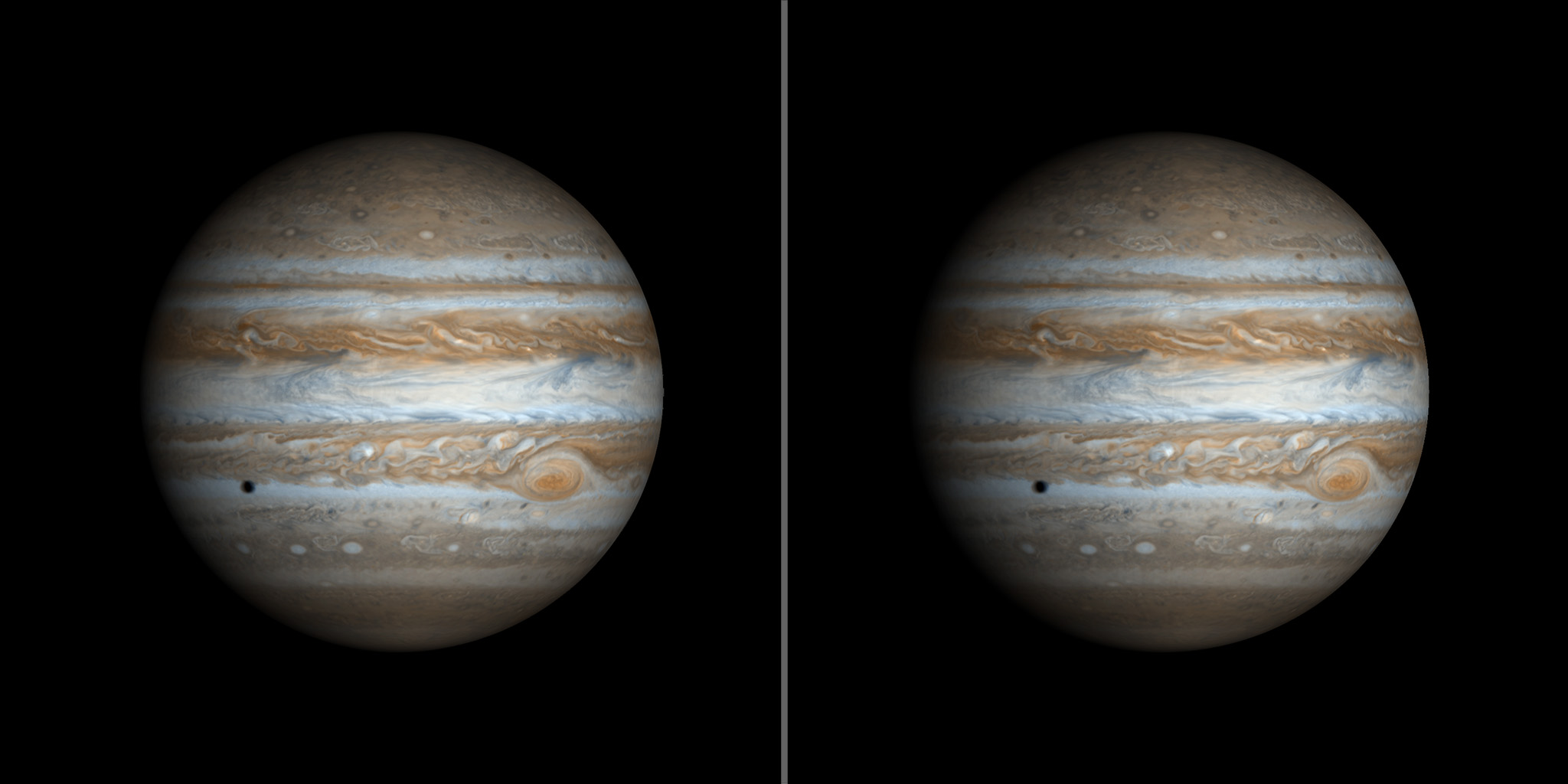
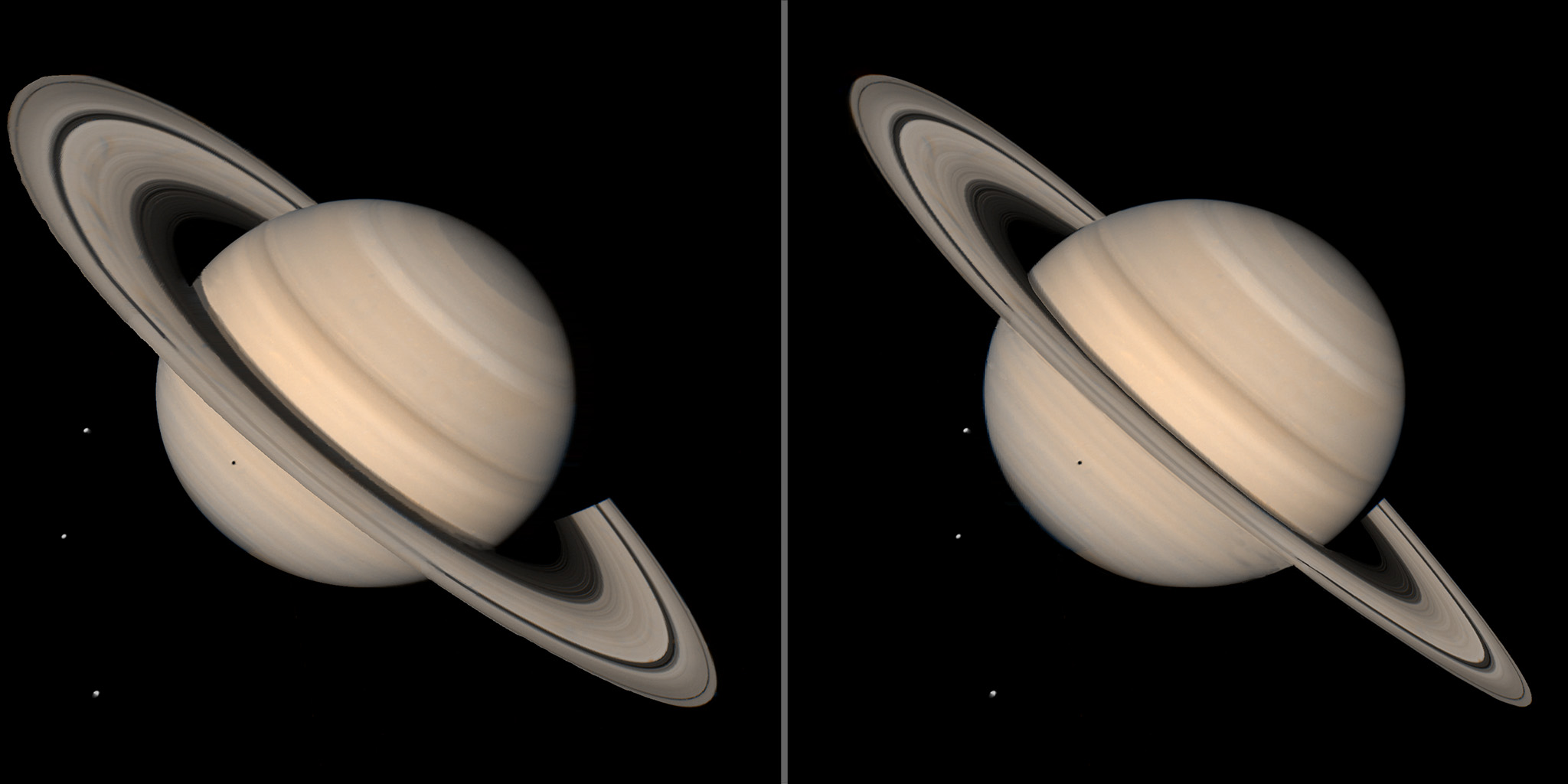
The following are the wall-eyed versions of them. Or return to the stereo index page.



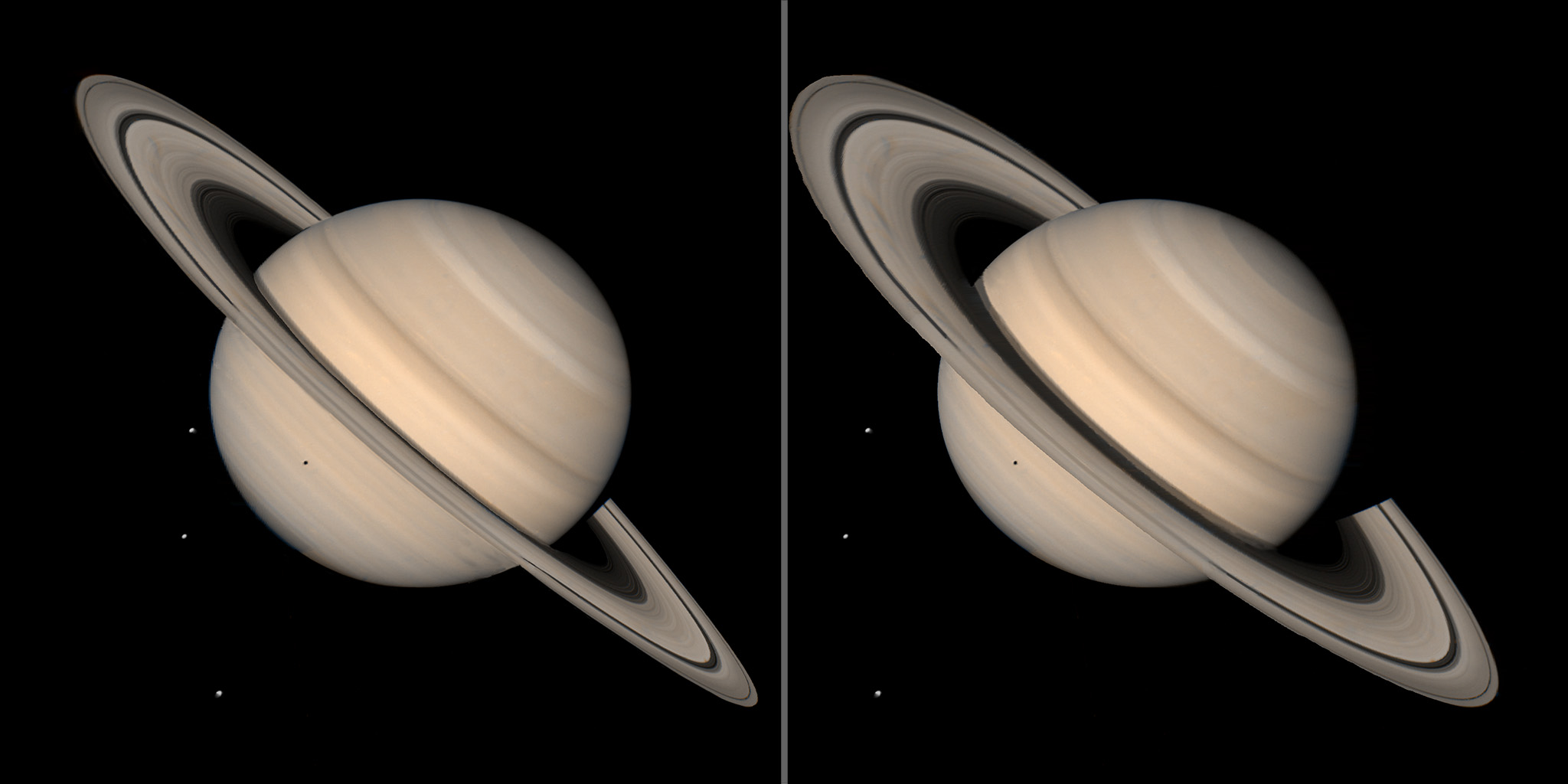
Return to the stereo index page.